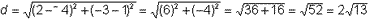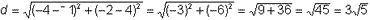Metric <-> Imperial <-> Metric
Cartesian Co-ordinates System
|
|
| Home | |
| Equivalents | |
| Internet Glossary |
Enter a coordinate pair for each the starting and ending points according to the Cartesian coodinate system. The script will calculate the straight line distance between the two points.
An Explanation of Cartesioan Co-ordinate System
|
Cartesian Coordinate System, Distance and Midpoint
Formula
|
||
|
The Cartesian Coordinate System consists of two
number lines perpendicular to each other at their 0's. The horizontal
axis is called the x-axis, and the vertical axis is called the y-axis.
This allows us to assign to each point in a plane a coordinate.
Each coordinate consists of a pair of numbers, the first of which
is the x-coordinate and the second is the y-coordinate, written
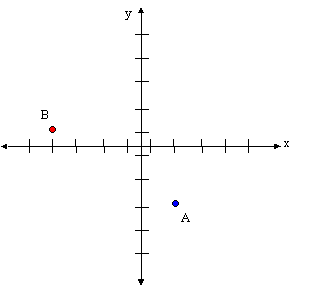
(x,y). For example, the point A (2,-3) is a point 2 units to the
right of the origin (the point (0,0)) and then 3 units down. And
point B (-4,1) is a point 4 units to the left of the origin and
then 1 unit up.
|
||
|
We
can find the distance between any two points in the xy-plane by
using a revised version of the Pythagorean Theorem called the distance
formula.
where d is the distance between the two points (xa,ya) and (xb,yb). Example 1: Find the distance between the points A and B given above.
Note: the order of the points A and B does not make a difference (try the above problem with the points switched).
We can also find the midpoint between these two points by taking the average of their coordinates. This translates into the following formula:
Example 2: Find the midpoint between the points A and B given above. midpoint
=
Example
3: Find the distance between point First we need to find the midpoint of segment BC. Now
we need to find the distance between this point Copyright ©1997 Bamdad Samii |